Recommendation for individuals using a screenreader: please set your punctuation settings to "most."
The resources below provide information about this test. The complete set of the competencies and
descriptive statements that define the content of the test—the test framework—is provided here.
Recommendation for individuals using a screenreader: please set your punctuation settings to "most."
Descriptive Statements:
- Demonstrate knowledge of place value and the relative magnitude of numbers.
- Use addition, subtraction, multiplication, and division of whole numbers in multidigit computations.
- Identify equivalent ways of representing integers, fractions, decimals, and percents, including the use of exponents and scientific notation.
- Apply operations with positive and negative integers, fractions, decimals, and percents.
- Solve word problems involving integers, fractions, decimals, percents, ratios, and proportions.
Sample Item:
The budget for a school district increased from 25.0 million dollars to 26.3 million dollars in one year. What was the percentage increase in the budget?
- 1.5%
- 4.9%
- 5.2%
- 13%
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
C. This question requires the examinee to solve word problems involving percents.
A change from 25.0 million dollars to 26.3 million dollars represents an increase of 1.3 million dollars (26.3 −minus 25.0 =equals 1.3). To find the percentage increase, divide 1.3 by 25.0 to get the decimal 0.052. Multiply the decimal result by 100 to convert it to a percentage (0.052 × 100 = 5.2%)(0.052 times 100 equals 5.2%).
Descriptive Statements:
- Evaluate algebraic expressions by substituting numbers for variables.
- Solve linear algebraic equations and inequalities in one variable.
- Identify equivalent algebraic expressions.
- Graph ordered pairs and number relationships presented in tabular or symbolic form.
- Identify the linear equation that best represents data presented in tabular or graphic form.
Sample Item:
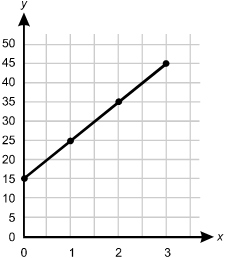
Axis X has values marked from 0 to 3 in increments of 1 marked at every 2nd grid line. Axis Y has values marked from 0 to 50 in increments of 5 marked at each grid line. The line has data points at (0, 15); (1, 25); (2, 35); and (3, 45).
The graph above shows the results of an experiment. Which of the following equations represents the graph?
- y = x + 10y equals x plus 10
- y = x + 15y equals x plus 15
- y = 10x + 15y equals 10x plus 15
- y = 15x + 10y equals 15x plus 10
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
C. This question requires the examinee to identify the linear equation that best represents data presented in graph form. The equation that represents a linear graph can be identified by using the equation y = mx + by equals mx plus b where m represents the slope of the line, and b represents the line's y-intercept. The slope of the line is the line's rise over the line's run and can be calculated using any two points on the line. Using the points (0, 15) and (1, 25), the rise can be found by subtracting 15 from 25 to get 10. The run can be found by subtracting 0 from 1 to get 1. The slope is therefore 10/110 over 1 or 10. The y-intercept is the y-coordinate of the point at which the line crosses the y-axis, which in the case of the given graph is 15. For the given line, the equation y = mx + by equals mx plus b can be rewritten y = 10x + 15y equals 10x plus 15.
Descriptive Statements:
- Identify a measurement or measurement unit needed to solve a problem.
- Convert units within and between standard and metric measurement systems.
- Solve problems involving lines, line segments, and angles.
- Analyze fundamental properties of triangles, quadrilaterals, and circles.
- Solve problems involving the length, perimeter, and area of basic shapes and the surface area and volume of rectangular solids.
- Solve real-world problems involving basic measurement and geometric concepts, including the Pythagorean theorem.
Sample Item:
It took a person 35 minutes to fix a problem on 6 computer workstations. If this person works at the same rate, how long will it take to fix the same problem on an additional 24 computers?
- 1 hour and 40 minutes
- 2 hours and 20 minutes
- 2 hours and 33 minutes
- 4 hours and 4 minutes
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
B. This question requires the examinee to convert units within measurement systems.
It takes 35 minutes to fix a problem on 6 workstations. To fix 24 workstations, 4 times as many, it must take 4 times as long, or 35 × 4 = 140 minutes35 times 4 equals 140 minutes. Two hours is 120 minutes, therefore 140 minutes must be 2 hours and 20 minutes.
Descriptive Statements:
- Calculate the probability of a given outcome.
- Analyze information presented in tables, line graphs, scatter plots, pictographs, bar graphs, histograms, and pie charts.
- Recognize the appropriate graphic representation of data.
- Compute and interpret the mean, median, and mode of data.
- Demonstrate knowledge of the concepts of range, standard deviation, and spread.
- Recognize appropriate and inappropriate uses of basic statistics.
Sample Item:
Summary data on the scores of four weekly quizzes are given below. Which week's quiz has the greatest spread in scores?
- Week 1: mean test score =equals 82%,
standard deviation =equals 12 points
- Week 2: mean test score =equals 78%,
standard deviation =equals 14 points
- Week 3: mean test score =equals 72%,
standard deviation =equals 10 points
- Week 4: mean test score =equals 61%,
standard deviation =equals 9 points
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
B. This question requires the examinee to demonstrate knowledge of the concept of spread. Standard deviation is a measure of the spread of data from the mean. Of the four choices, choice B has the greatest standard deviation and therefore the greatest spread.
Descriptive Statements:
- Estimate the solution to a given problem.
- Evaluate the reasonableness of a solution to a given computation or problem.
- Use algorithms (i.e., a set of instructions) to perform a given calculation or solve a given problem.
- Use inductive reasoning to identify missing terms in numerical and graphical patterns.
- Use deductive reasoning to draw conclusions and evaluate arguments.
- Translate between written English and mathematical terminology, concepts, and notation.
Sample Item:
The surface area of the earth is roughly 1.97 × 1081.97 times 10 to the power of 8 square miles and the population of the earth is about 6.5 × 1096.5 times 10 to the power of 9 people. Most of the earth's surface is water—only about 30% of the earth's surface is land. Given this information, which of the following is the best estimate of the average number of people per square mile of the earth's land surface?
- approximately 10
- approximately 30
- approximately 100
- approximately 300
Correct Response and Explanation (Show Correct ResponseHide Correct Response)
C. This question requires the examinee to estimate the solution to a given problem. To solve this problem, begin by rounding 1.97 to 2.0 and rounding 6.5 to 6.0. Since 30% of the earth's surface is land, an estimate of the number of square miles of land on the earth would be 30% of 2.0 × 1082.0 times 10 to the power of 8 square miles or 0.6 × 1080.6 times 10 to the power of 8 square miles. The number of people per square mile can be estimated by dividing 6.0 × 1096.0 times 10 to the power of 9 people by 0.6 × 1080.6 times 10 to the power of 8 square miles or the equivalent 6.0 × 1076.0 times 10 to the power of 7 square miles. 6.0 × 1096.0 times 10 to the power of 9 people divided by 6.0 × 1076.0 times 10 to the power of 7 square miles is 100 people per square mile.

